A graph
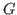
is called fractional
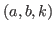
-critical covered if

is fractional
![$[a,b]$](img54.png)
-covered for any
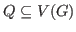
with

. In this article, we demonstrate an
independence number and minimum degree condition for a graph to be fractional
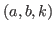
-critical
covered, which is a generalization of Zhou's previous result [S. Zhou, Some new sufficient
conditions for graphs to have fractional
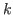
-factors, International Journal of Computer
Mathematics 88(3)(2011)484-490]. Furthermore, we demonstrate that two conditions on independence
number and minimum degree in our main result are sharp.
-covered graph, fractional
-critical covered graph.