Next: Aurelian Cernea: An existence Up: TOME 51 (99), no. Previous: Miruna Beldiman, Eugenia Panaitescu
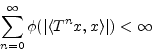
In the continuous case we prove that a strongly continuous uniformly
bounded semigroup of operators acting on a Hilbert space ![]() is
spectrally stable (i.e. the spectrum of its infinitesimal generator
lies in the open left half plane) if and only if for each
is
spectrally stable (i.e. the spectrum of its infinitesimal generator
lies in the open left half plane) if and only if for each ![]() and each
and each
![]() one has:
one has:
Key Words: Spectral radius, discrete semigroups, strongly continuous semigroups, uniform exponential stability, Orlicz space.
2000 Mathematics Subject Classification: Primary: 47D03,
Secondary: 11M35.
Download the paper in pdf format here.