Arturas Dubickas: On the approximation of the Thue-Morse generating sequence, p.59-71

Abstract:

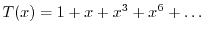
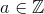
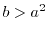
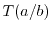
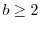
Let $T(x)=1+x+x^3+x^6+\dots$ be the generating function of the Thue-Morse sequence. We show that for any coprime nonzero integers $a \in \Z$ and $b \in \N$ satisfying $b>a^2$ the irrationality exponent of $T(a/b)$ does not exceed $(2\log b-2\log \vert a\vert)/(\log b-2\log \vert a\vert)$. 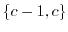We also prove that infinitely many partial quotients of the number $T(\pm 1/b)$, where $b \geq 2$ is an integer, lie in the set $\{c-1, c\}$ for some integer $c=c(\pm 1,b) \geq 2$. 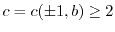For instance, the continued fraction of $T(-1/3)$ has infinitely many partial quotients smaller than or equal to $3$. 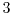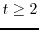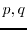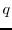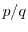In passing, we obtain the following Lagrange type result: if for an irrational number $\al$ whose continued fraction expansion has only finitely many partial quotients smaller than or equal to $t-1$, where $t \geq 2$ is an integer, and some coprime integers $p, q$, where $q$ is large enough, we have |α - p/q| < (t - 1)/tq² then $p/q$ is a convergent to α.

Key Words: Thue-Morse sequence, irrationality measure, continued fraction, Lagrange's theorem.

2000 Mathematics Subject Classification: Primary: 11J04;
Secondary: 11J70, 11J82.

Download the paper in pdf format here.